
The resistance to deformation in a polymer comes from entanglement, including both physical crosslinks and more general occlusions as chains encounter each other while undergoing conformational changes to accommodate the new shape of the material. Why would energy be lost in this experiment? In a polymer, it has to do chiefly with chain flow. It measures energy lost during that cycling strain. The difference between the loading and unloading curves is called the loss modulus, E". The storage modulus is a measure of how much energy must be put into the sample in order to distort it.
The slope of the loading curve, analogous to Young's modulus in a tensile testing experiment, is called the storage modulus, E'. When the sample snapped back the same distance, the force was unequal to the one that was initially applied. A force was applied to move a sample or a portion of a sample, some distance. The difference between the loading curve (when the stress was first applied) and the unloading curve (when the stress was removed) represents an energy loss. Again, we can see this in the curve below, where the curvature has been exaggerated. As we let the sample relax back to its initial length, the strain is different from what we saw when we were stretching it.
However, it depends whether we are stretching the sample or letting it relax again. In this case, Hooke's Law seems to imply that a specific sample subjected to a specific strain would experience a specific stress (or vice versa). Hysteresis just means that a property of the material depends on how the material came to be in its current situation. Instead, there is a phenomenon called hysteresis at work. In the picture below, the curvature is exaggerated quite a bit, just for illustrative purposes.Įven if the relationship is not quite linear, then as we release the strain, the stress in the material should simply follow the curve back down to zero. In reality, even within the linear elastic region, the stress-strain curve is not quite linear. We can get this information because polymers don't quite follow Hooke's Law perfectly. The principle reason for running the experiment this way is to get some additional information. Now, one experiment should be good enough to extract the modulus, but we are letting go and doing it over again. Instead of continuously moving all the way through the linear elastic region, beyond which Hooke's law breaks down, we carefully keep the sample in the Hookean region for the entire experiment. We can use dynamic mechanical analysis to measure the modulus of the material. This approach is called dynamic mechanical analysis. Instead of a continuously increasing strain, this sample is subjected to an oscillatory strain, one that repeats in a cycle. We can then stress it again and release it again. Instead of stretching the material as far as we can, we will only stretch it a tiny bit, then release the stress so that it snaps back to its original length. Now we will look at a much more limited approach. We continued to stretch the material farther and farther, applying generally increasing stress until the material finally broke. In the experiments we saw earlier, we didn't let go. The same force is what snaps the spring back into place once you let it go.
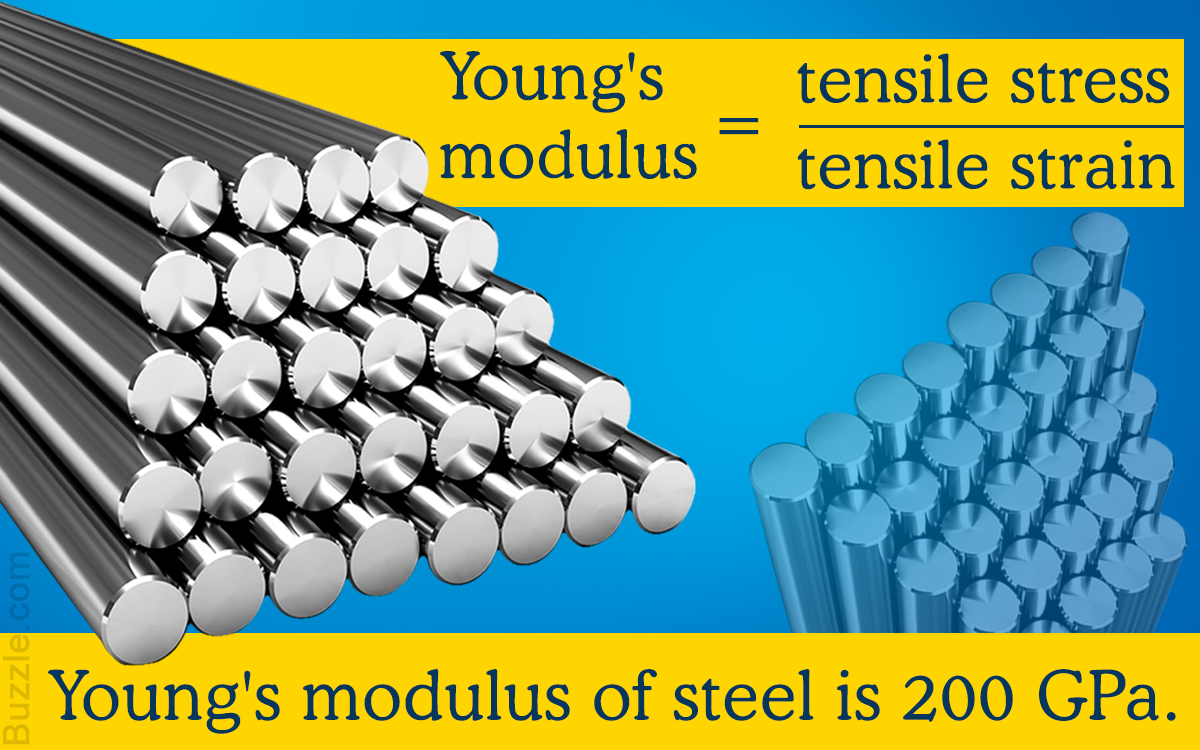
The modulus can be thought of the resistance to stretching a spring the more resistance the spring offers, the greater the force needed to stretch it. Hooke's Law is sometimes used to describe the behavior of mechanical springs. That's the proportionality constant between stress and strain in Hooke's Law. If we graph the relationship, then the slope of the line gives us Young's modulus, E. If the strain is limited to a very small deformation, then it varies linearly with stress. The stress is the force exerted on the sample divided by the cross-sectional area of the sample. The strain is the amount of deformation in the material, such as the change in length in an extensional experiment, expressed as a fraction of the beginning length. The Young's modulus is the ratio of the stress-induced in a material under an applied strain. We saw earlier that the inherent stiffness of a material can be assessed by its Young's modulus.


 0 kommentar(er)
0 kommentar(er)
